はじめに
Matplotlibライブラリを利用して、を作成します。
【前の内容】
【目次】
傾いた3次元格子の作図
前回は、Matplotlibライブラリを利用して、3次元空間上に直方体の格子のグラフを作成します。
今回は、3次元空間上に平行六面体の格子のグラフを作成します。前回と「2次元格子の作図【Matplotlib】 - からっぽのしょこ」の記事も参照してください。
利用するライブラリを読み込みます。
# 利用ライブラリ import numpy as np import matplotlib.pyplot as plt from matplotlib.animation import FuncAnimation
3次元格子の描画
3次元の格子(平行六面体の立体)の作図方法を確認します。
x軸・y軸・z軸の値を作成します。(説明に数式を使いますが、特に重要なわけではないので読み飛ばしてください。)
# 点の座標を指定 p = np.array([1.0, 1.0, 1.0]) q = np.array([1.0, -1.0, -1.0]) r = np.array([-1.0, 1.0, -1.0]) # 立体用の係数を指定 a = np.arange(start=0.0, stop=4.1, step=1.0) b = np.arange(start=0.0, stop=3.1, step=1.0) c = np.arange(start=0.0, stop=2.1, step=1.0) print(a) print(b) print(c) print(a.shape) print(b.shape) print(c.shape) # 係数の格子点を作成 A, B, C = np.meshgrid(a, b, c) print(A.shape) print(B.shape) # 立体の座標を計算 X = A * p[0] + B * q[0] + C * r[0] Y = A * p[1] + B * q[1] + C * r[1] Z = A * p[2] + B * q[2] + C * r[2] print(X[0]) print(Y[0]) print(Z[0])
[0. 1. 2. 3. 4.]
[0. 1. 2. 3.]
[0. 1. 2.]
(5,)
(4,)
(3,)
(4, 5, 3)
(4, 5, 3)
[[ 0. -1. -2.]
[ 1. 0. -1.]
[ 2. 1. 0.]
[ 3. 2. 1.]
[ 4. 3. 2.]]
[[0. 1. 2.]
[1. 2. 3.]
[2. 3. 4.]
[3. 4. 5.]
[4. 5. 6.]]
[[ 0. -1. -2.]
[ 1. 0. -1.]
[ 2. 1. 0.]
[ 3. 2. 1.]
[ 4. 3. 2.]]
点・点
・点
の座標(x軸の値
・y軸の値
・z軸の値
)を
p, q, rとして指定します。Pythonのインデックスに合わせて、添字を0から割り当てています。
立体の座標計算に使う係数の値を
a, b, cとして作成して、格子状の点(全ての組み合わせ)をnp.meshgrid()で作成してA, B, Cとします。
平面のx軸の値・y軸の値
・z軸の値
を計算して、
X, Y, Zとします。
X, Y, Zを用いて、散布図を作成してプロット位置を確認します。
# 3D格子点を作図 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p.round(2)))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q.round(2)))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r.round(2)))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') fig.suptitle('Axes.scatter(X, Y, Z)', fontsize=20) ax.legend() ax.set_aspect('equal') #ax.view_init(elev=90, azim=270) # xy面 #ax.view_init(elev=0, azim=270) # xz面 #ax.view_init(elev=0, azim=0) # yz面 plt.show()
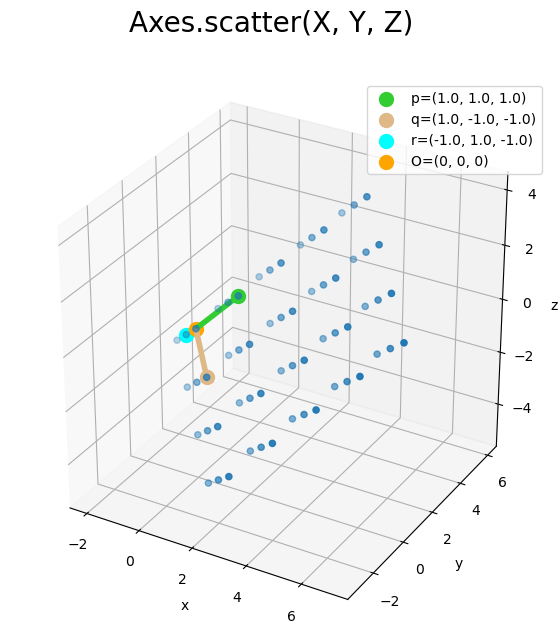
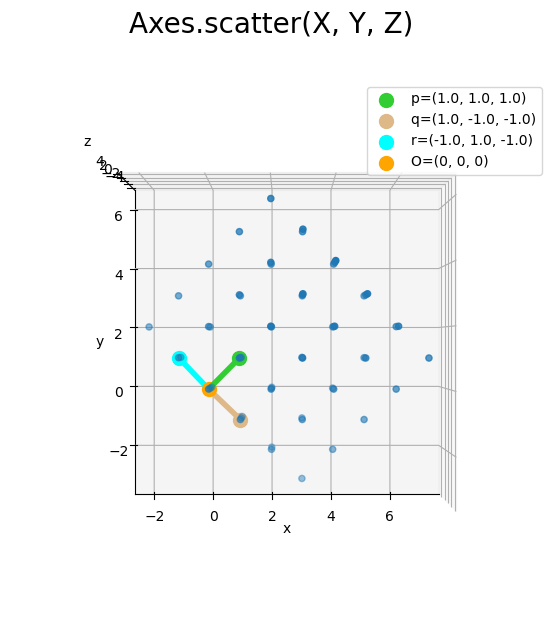
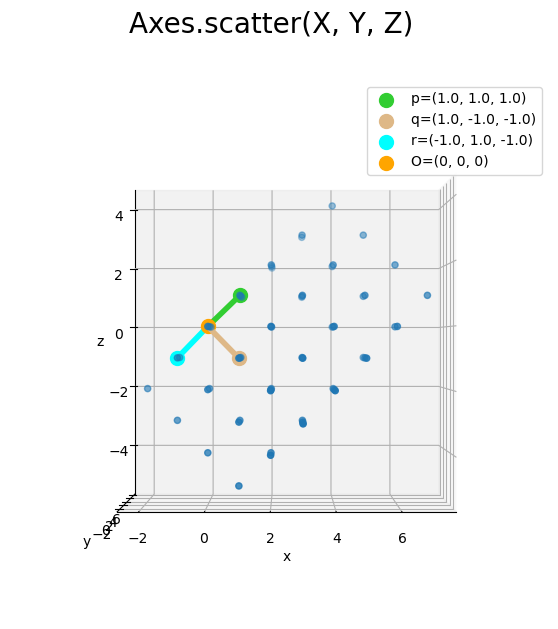
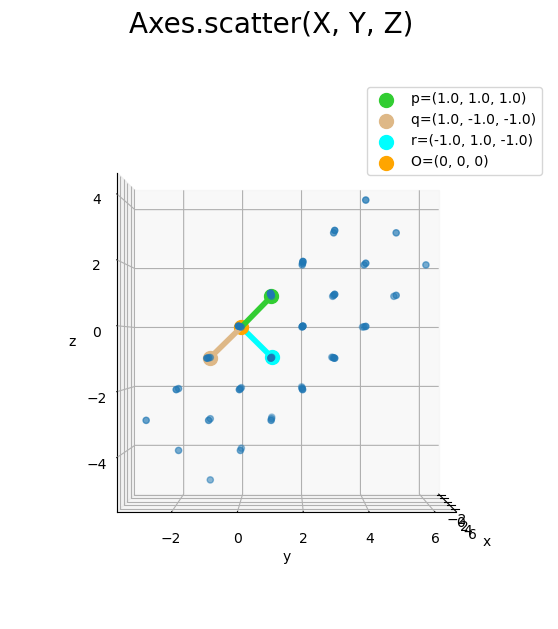
対角線方向にそれぞれ等間隔の点が平行六面体に並びます。面ごとに見ると平行四辺形に並びます。傾きは基準となる点p, q, r、間隔と個数は係数a, b, cによって決まります。
原点(オレンジ色の点)からx軸方向にp[0], q[0], r[0]、y軸方向にp[1], q[1], r[1]、z軸方向にp[2], q[2], r[2]移動した点が点p(黄緑色の点)・点q(ベージュ色の点)・点r(水色の点)です。
X, Y, Zの1つ目の次元(0番目の軸)に関して、for文で繰り返し平面を描画します。
# 平面を作図 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p.round(2)))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q.round(2)))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r.round(2)))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 for i in range(len(b)): ax.plot_wireframe(X[i], Y[i], Z[i], alpha=0.5) # p,rに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') fig.suptitle('Axes.plot_wireframe(X[i], Y[i], Z[i])', fontsize=20) ax.legend() ax.set_aspect('equal') #ax.view_init(elev=0, azim=270) # xz面 plt.show()
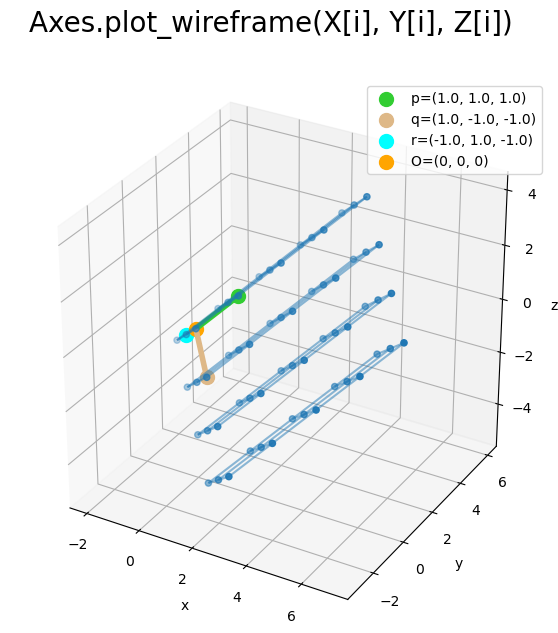
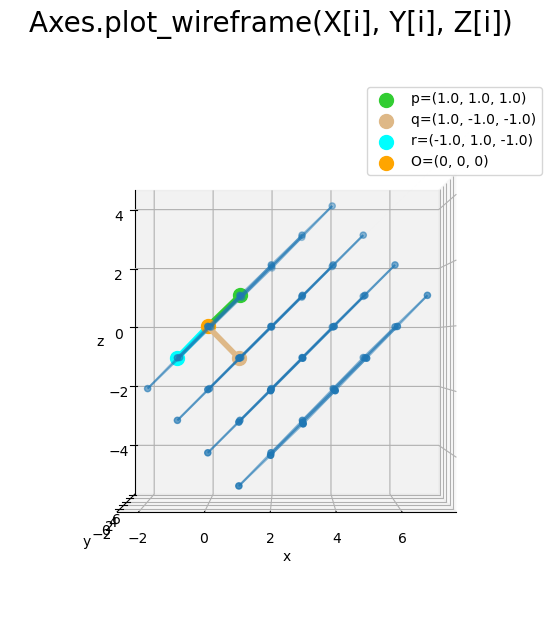
bの要素数(X, Y, Zの0番目の軸の要素数len(X)やX.shape[0])に応じて、原点と点p, rを通る平面と平行な平面です。
同様に、X, Y, Zの2つ目の次元(1番目の軸)に関して平面を描画します。
# 平面を作図 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p.round(2)))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q.round(2)))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r.round(2)))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 for i in range(len(a)): ax.plot_wireframe(X[:, i], Y[:, i], Z[:, i], alpha=0.5) # q,rに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') fig.suptitle('Axes.plot_wireframe(X[:, i], Y[:, i], Z[:, i])', fontsize=20) ax.legend() ax.set_aspect('equal') #ax.view_init(elev=90, azim=270) # xy面 plt.show()
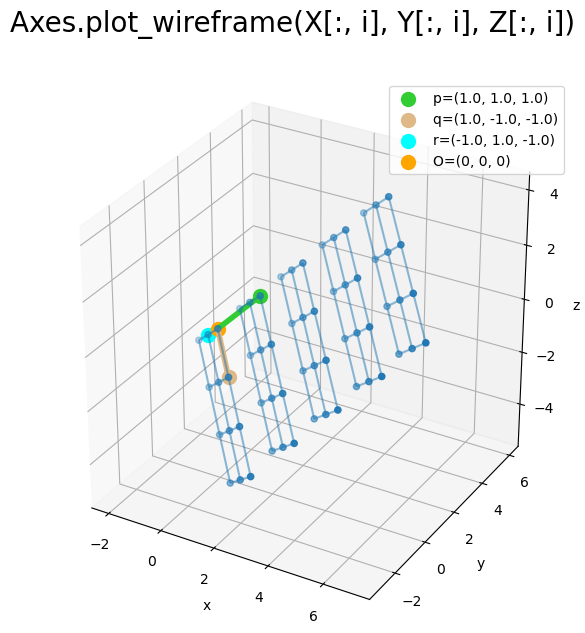
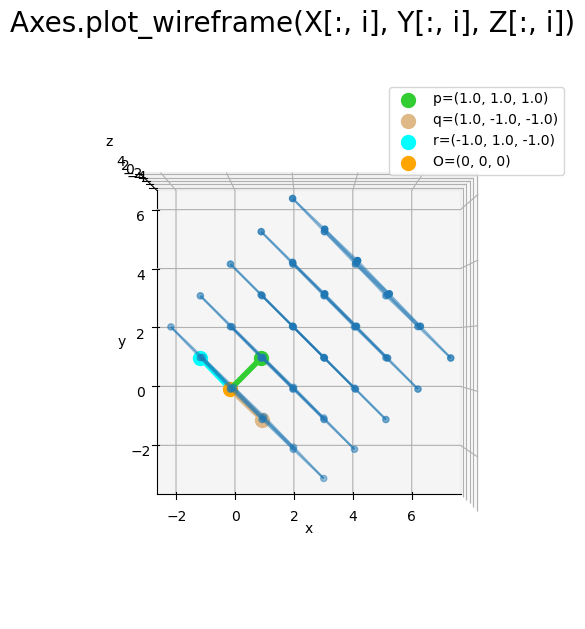
aの要素数(X, Y, Zの1番目の軸の要素数len(X[0])やX.shape[1])に応じて、原点と点q, rを通る平面と平行な平面です。
X, Y, Zの3つ目の次元(2番目の軸)に関して平面を描画します。
# 平面を作図 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p.round(2)))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q.round(2)))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r.round(2)))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 for i in range(len(c)): ax.plot_wireframe(X[:, :, i], Y[:, :, i], Z[:, :, i], alpha=0.5) # p,qに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') fig.suptitle('Axes.plot_wireframe(X[:, :, i], Y[:, :, i], Z[:, :, i])', fontsize=20) ax.legend() ax.set_aspect('equal') #ax.view_init(elev=0, azim=0) # yz面 plt.show()
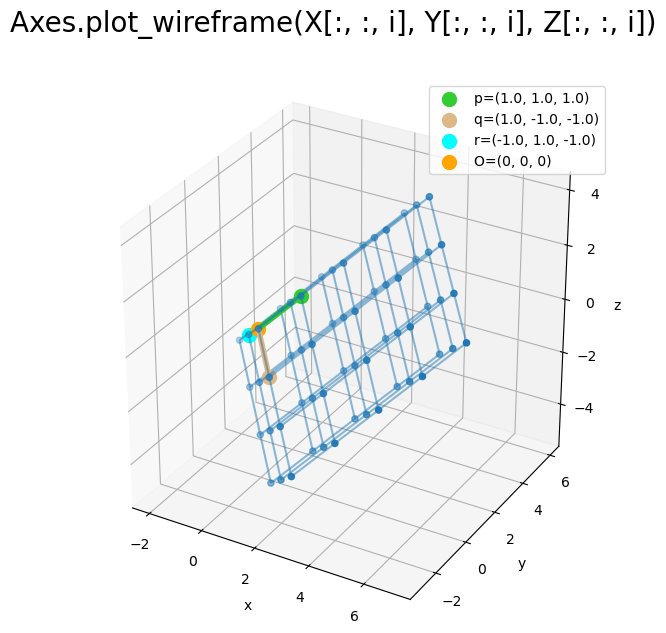
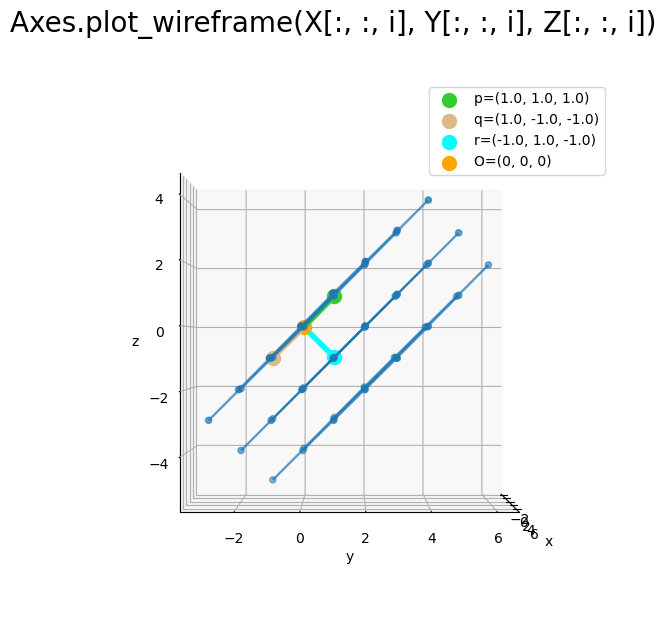
cの要素数(X, Y, Zの2つ目の軸の要素数len(X[0, 0])やX.shape[2])に応じて、原点と点p, qを通る平面と平行な平面です。
X, Y, Zの全ての次元に関してそれぞれ平面を描画します。
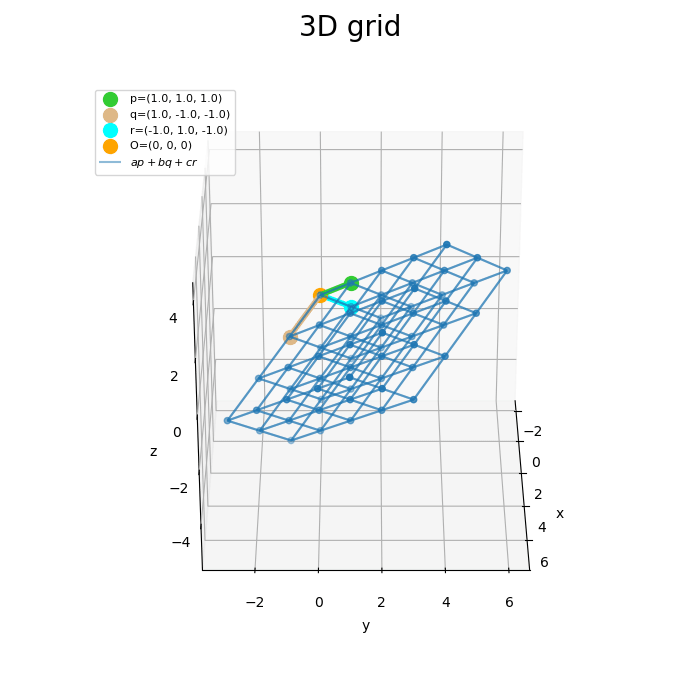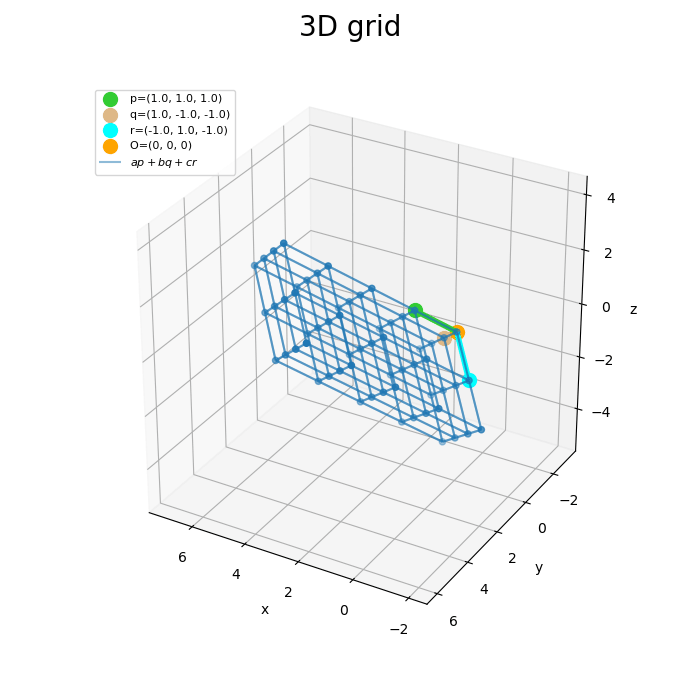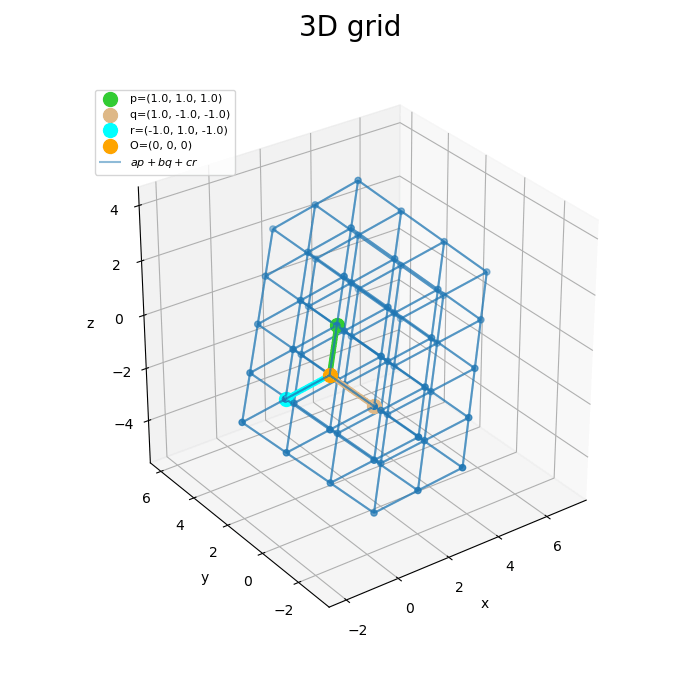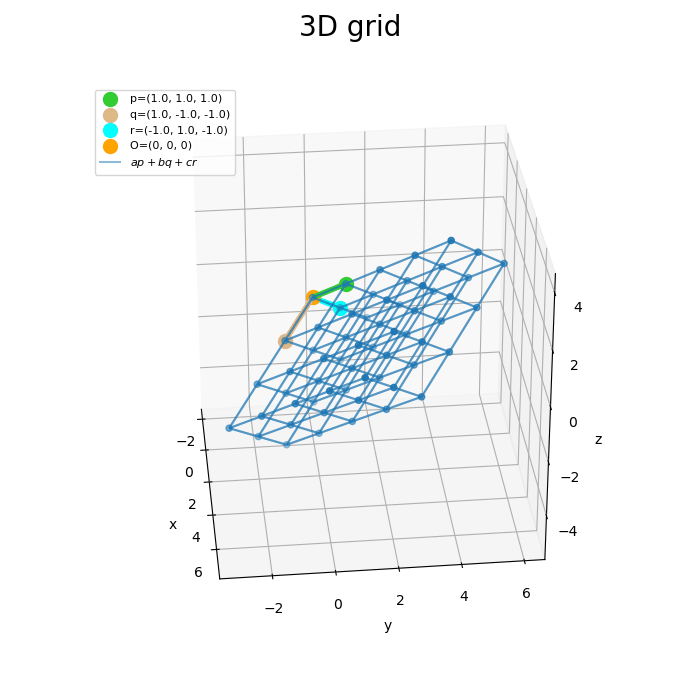
# 3D格子を作図 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p.round(2)))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q.round(2)))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r.round(2)))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 for i in range(len(b)): # 凡例用に1つだけラベルを設定 if i == 0: label_text = '$a p + b q + c r$' else: label_text = '' ax.plot_wireframe(X[i], Y[i], Z[i], alpha=0.5, label=label_text) # p,rに関する平面 for i in range(len(a)): ax.plot_wireframe(X[:, i], Y[:, i], Z[:, i], alpha=0.5) # q,rに関する平面 for i in range(len(c)): ax.plot_wireframe(X[:, :, i], Y[:, :, i], Z[:, :, i], alpha=0.5) # p,qに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') fig.suptitle('3D grid', fontsize=20) ax.legend() ax.set_aspect('equal') #ax.view_init(elev=90, azim=270) # xy面 #ax.view_init(elev=0, azim=270) # xz面 #ax.view_init(elev=0, azim=0) # yz面 plt.show()
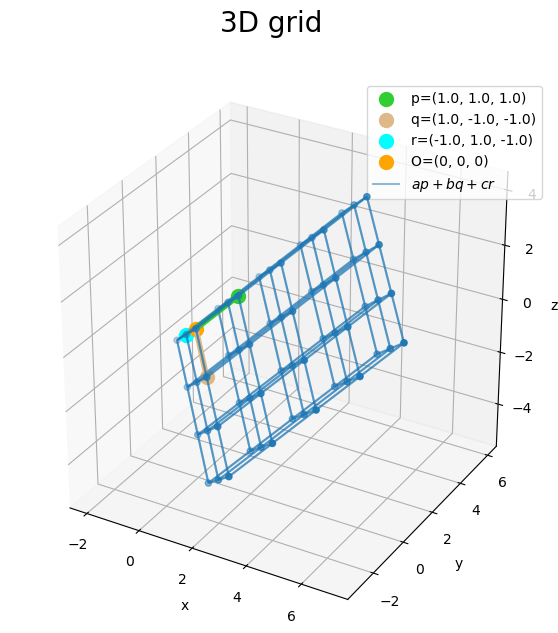
3方向の平面を組み合わせることで、3次元格子を描画できました。
原点とp, q, rそれぞれを結ぶ直線と平行な直線で立体が構成されるので、2方向の面を組み合わせれば、3次元格子を描画できます。
凡例を1つだけ表示するために、1つ目の平面の描画時のみlabel引数に文字列を指定します。if文を使って、初回(iが0のとき)以外は空の文字列''を指定します。
グラフを回転させて確認します。
・作図コード(クリックで展開)
# フレーム数を指定 frame_num = 60 # 水平方向の角度として利用する値を作成 h_n = np.linspace(start=0.0, stop=360.0, num=frame_num+1)[:frame_num] # グラフオブジェクトを初期化 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) fig.suptitle('3D grid', fontsize=20) # 作図処理を関数として定義 def update(i): # 前フレームのグラフを初期化 plt.cla() # i番目の角度を取得 h = h_n[i] # 3D格子を作図 ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 for i in range(len(b)): # 凡例用に1つだけラベルを設定 label_text = '$a p + b q + c r$' if i == 0 else '' ax.plot_wireframe(X[i], Y[i], Z[i], alpha=0.5, label=label_text) # p,rに関する平面 for i in range(len(a)): ax.plot_wireframe(X[:, i], Y[:, i], Z[:, i], alpha=0.5) # q,rに関する平面 for i in range(len(c)): ax.plot_wireframe(X[:, :, i], Y[:, :, i], Z[:, :, i], alpha=0.5) # p,qに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') ax.legend(loc='upper left', prop={'size': 8}) ax.set_aspect('equal') ax.view_init(elev=30, azim=h) # 表示角度 # gif画像を作成 ani = FuncAnimation(fig=fig, func=update, frames=frame_num, interval=100) # gif画像を保存 ani.save('solid360_3d.gif')
作図処理をupdate()として定義して、FuncAnimation()でgif画像を作成します。
if文を1行処理する記法を使っています。
基準となる3点の値を変化させたアニメーションを作成します。
・作図コード(クリックで展開)
# フレーム数を指定 frame_num = 60 # 固定する点の座標を指定 p = np.array([1.0, 1.0, 1.0]) q = np.array([1.0, -1.0, -1.0]) # 変化する点の座標用の値(ラジアン)を作成 t_n = np.linspace(start=0.0, stop=2.0*np.pi, num=frame_num+1)[:frame_num] u_n = np.linspace(start=1.0/3.0*np.pi, stop=1.0/3.0*np.pi, num=frame_num+1)[:frame_num] # グラフオブジェクトを初期化 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) fig.suptitle('3D grid', fontsize=20) # 作図処理を関数として定義 def update(i): # 前フレームのグラフを初期化 plt.cla() # i番目の値を取得 t = t_n[i] u = u_n[i] # 変化する点の座標を作成 r = np.array([np.sin(t)*np.cos(u), np.sin(t)*np.sin(u), np.cos(t)]) # 立体の座標を計算 X = A * p[0] + B * q[0] + C * r[0] Y = A * p[1] + B * q[1] + C * r[1] Z = A * p[2] + B * q[2] + C * r[2] # 3D格子を作図 ax.scatter(X, Y, Z) # 格子点 ax.quiver(0, 0, 0, p[0], p[1], p[2], color='limegreen', linewidth=4, arrow_length_ratio=0.0) # pに関する直線 ax.quiver(0, 0, 0, q[0], q[1], q[2], color='burlywood', linewidth=4, arrow_length_ratio=0.0) # qに関する直線 ax.quiver(0, 0, 0, r[0], r[1], r[2], color='aqua', linewidth=4, arrow_length_ratio=0.0) # rに関する直線 ax.scatter(p[0], p[1], p[2], color='limegreen', s=100, label='p=('+', '.join(map(str, p.round(2)))+')') # 点p ax.scatter(q[0], q[1], q[2], color='burlywood', s=100, label='q=('+', '.join(map(str, q.round(2)))+')') # 点q ax.scatter(r[0], r[1], r[2], color='aqua', s=100, label='r=('+', '.join(map(str, r.round(2)))+')') # 点r ax.scatter(0, 0, 0, color='orange', s=100, label='O=(0, 0, 0)') # 原点 for i in range(len(b)): # 凡例用に1つだけラベルを設定 label_text = '$a p + b q + c r$' if i == 0 else '' ax.plot_wireframe(X[i], Y[i], Z[i], alpha=0.5, label=label_text) # p,rに関する平面 for i in range(len(a)): ax.plot_wireframe(X[:, i], Y[:, i], Z[:, i], alpha=0.5) # q,rに関する平面 for i in range(len(c)): ax.plot_wireframe(X[:, :, i], Y[:, :, i], Z[:, :, i], alpha=0.5) # p,qに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') ax.legend(loc='upper left', prop={'size': 8}) ax.set_xlim(left=-1.0, right=8.0) ax.set_ylim(bottom=-6.0, top=6.0) ax.set_zlim(bottom=-5.0, top=6.0) ax.set_aspect('equal') # gif画像を作成 ani = FuncAnimation(fig=fig, func=update, frames=frame_num, interval=100) # gif画像を保存 ani.save('solid_3d.gif')
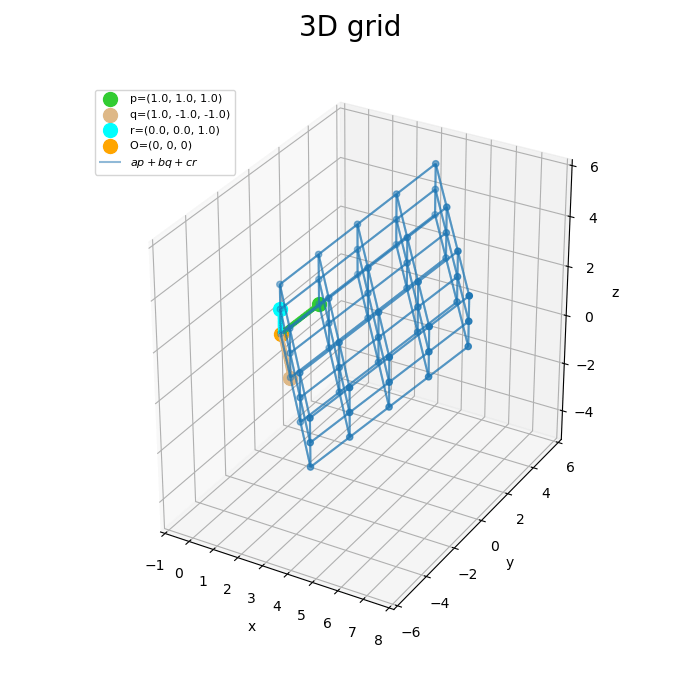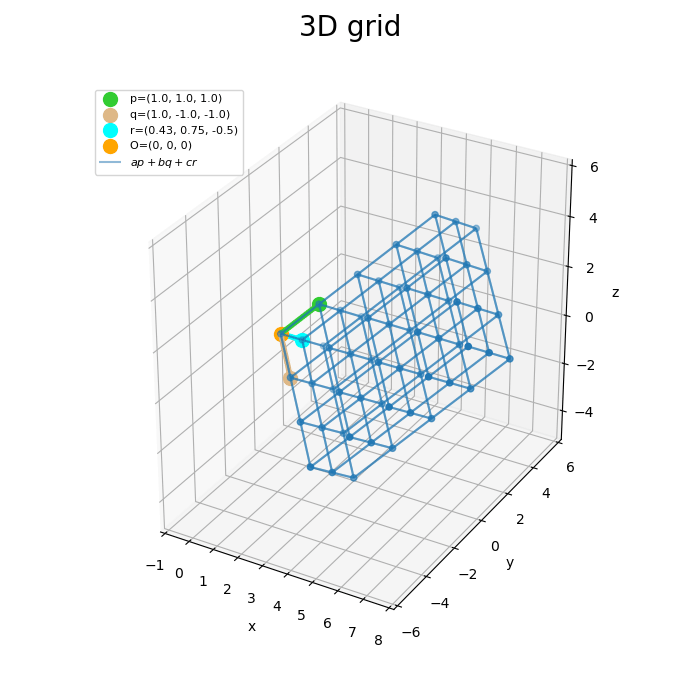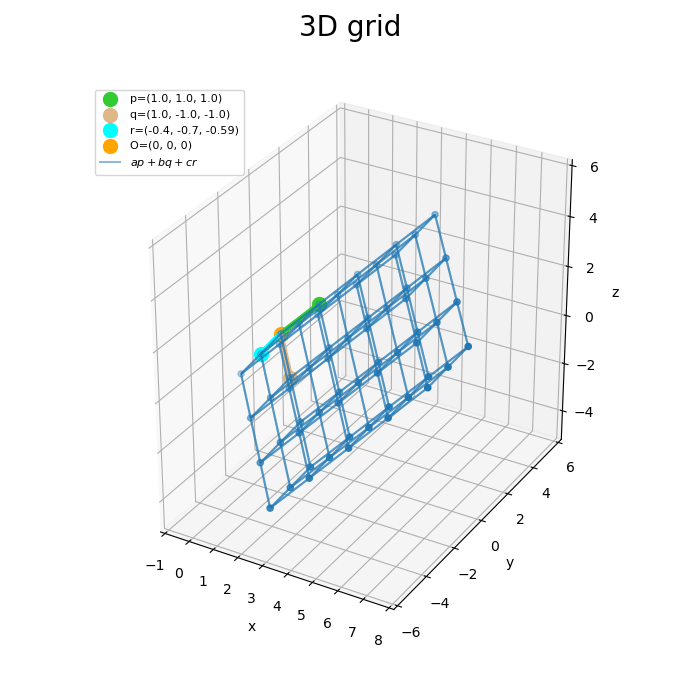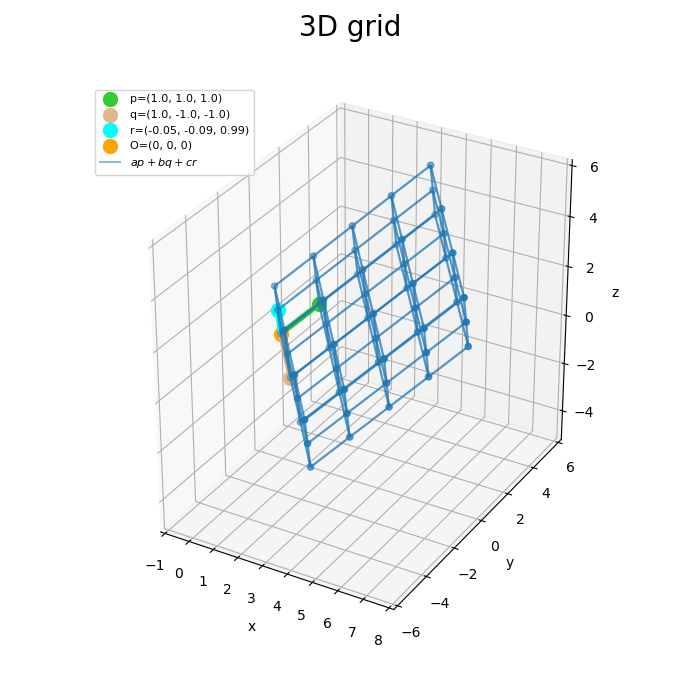
この例では、点rが単位球面上を移動し、それに応じて格子の形(平行六面体の立体)が変化します。
3次元配列X, Y, Zの全ての次元を(1次元配列x, y, zを)同じ要素数にしておくと、1つのfor文で処理できます。
# 点の座標を指定 p = np.array([1.0, 1.0, 1.0]) q = np.array([1.0, -1.0, -1.0]) r = np.array([-1.0, 1.0, -1.0]) # 立体用の係数を指定 a = np.arange(start=-2.0, stop=2.1, step=2.0) print(a.shape) # 係数の格子点を作成 A, B, C = np.meshgrid(a, a, a) print(A.shape) print(B.shape) print(C.shape) # 立体の座標を計算 X = A * p[0] + B * q[0] + C * r[0] Y = A * p[1] + B * q[1] + C * r[1] Z = A * p[2] + B * q[2] + C * r[2] # 3D格子を作図 fig, ax = plt.subplots(figsize=(7, 7), facecolor='white', subplot_kw={'projection': '3d'}) for i in range(len(X)): # 凡例用に1つだけラベルを設定 label_text = '$a p + b q + c r$' if i == 0 else '' ax.plot_wireframe(X[i], Y[i], Z[i], label=label_text) # p,rに関する平面 ax.plot_wireframe(X[:, i], Y[:, i], Z[:, i]) # q,rに関する平面 ax.plot_wireframe(X[:, :, i], Y[:, :, i], Z[:, :, i]) # p,qに関する平面 ax.set_xlabel('x') ax.set_ylabel('y') ax.set_zlabel('z') fig.suptitle('3D grid', fontsize=20) ax.legend() ax.set_aspect('equal') #ax.view_init(elev=90, azim=270) # xy面 #ax.view_init(elev=0, azim=270) # xz面 #ax.view_init(elev=0, azim=0) # yz面 plt.show()
(3,)
(3, 3, 3)
(3, 3, 3)
(3, 3, 3)
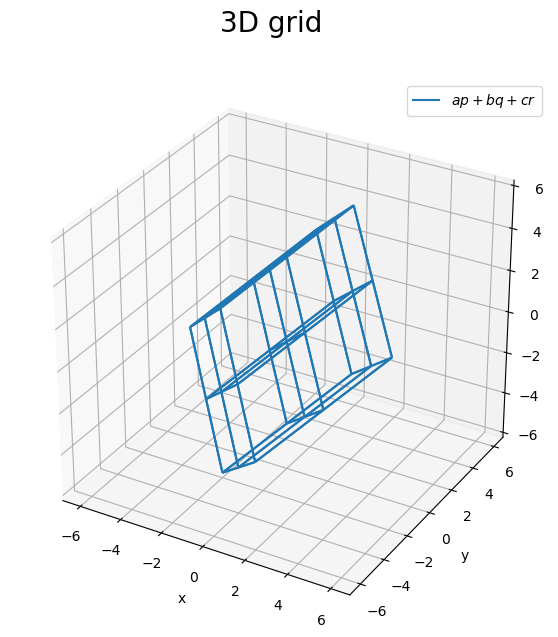
以上で、傾いた3次元の格子のグラフを作成します。次は、円のグラフを作成します。
おわりに
これで格子編(なんだそりゃ)が完成です。次は円編です。
【次の内容】